In order to simplify the analysis, there are some considerations that should be taken before proceeding with the calculations,
1
Initial considerations:
The human
body has been divided in four groups:
o
The first
group is formed by the feet, which are considered immobile and serve inertial
reference system for the entire Sit-to-stand movement.
o
The second
group corresponds to the legs, which rotate on reference of the feet with
ankle’s axis.
o
The third
group is made up of the thighs, which rotate on reference of the legs with
knee’s axis and finally.
o
The fourth
group, which, for reasons of simplifying calculations, consider together the
trunk, head and upper limbs. This rotates on reference of the third element
(thighs) with knee’s axis with hip’s axis.
The relative
parameters: weight, center of gravity, location and radius of gyration, which
have been used for this work, are based on anthropomorphic models, obtained
from samples of body parts, made by Dempster-Winter (1955, amended in 2009) and
Zatsiorsky-Seluyanov, (1996, amended in 2002). See Table 02.
Segment
|
Center of gravity (%)
|
Relative Weight (%)
|
Radius of gyration
Kxx
|
Radius of gyration
Kyy
|
Radius of gyration
Kzz
|
Head and neck
|
60.4
|
0.0694
|
0.362
|
0.312
|
0.376
|
Trunk
|
49.5
|
0.4346
|
0.372
|
0.191
|
0.347
|
Arm
|
43.6
|
0.0271
|
0.285
|
0.158
|
0.269
|
Forearm
|
43
|
0.0162
|
0.276
|
0.121
|
0.265
|
Hand
|
50.6
|
0.0061
|
0.628
|
0.401
|
0.513
|
Thigh
|
43.3
|
0.1416
|
0.329
|
0.149
|
0.329
|
Leg
|
43.3
|
0.0433
|
0.251
|
0.102
|
0.246
|
Foot
|
42.9
|
0.0137
|
0.257
|
0.124
|
0.245
|
TABLE 02: Human body parameters
2
Method of Lagrange – EULER
formulation
2.1
Lagrange -equation
$$L=\sum { { E }c_{ i }- } \sum { { E }p_{ i } } $$
$$\sum { { E }c_{ i } } =\frac { 1 }{ 2 } \sum _{ i }^{ n }{ \left[ \overline { v } _{ k }^{ T }{ m }_{ k }{ \overline { v } }_{ k }+\overline { w } _{ k }^{ T }{ D }_{ k }{ \overline { w } }_{ k } \right] } $$$$\sum { { E }p_{ i } } =-\sum _{ i }^{ n }{ \left[ { m }_{ k }\overline { g } ^{ T }{ \overline { C } }_{ k } \right] } $$
Where:
|
|
vk:
|
Translational speed of the
k-th element
|
wk:
|
Rotational speed of the k-th
element
|
mk:
|
Mass of the k-th element
|
Dk:
|
Inertia tensor of the k-th
element regarding X0Y0Z0 and moved to its
center of mass..
|
Ck:
|
Center of mass of the k-th
element
|
g:
|
Gravity
|
3 Method of Newton – EULER formulation
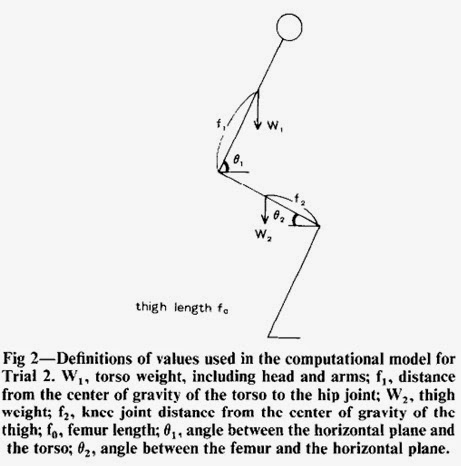
o The θ1, θ2, θ3, angles were taken on reference of the horizontal axis.o Each element is taken as a rigid body.
o Motion of various part of the body occur in a vector plane so that rotations of the body may be disregarded.
o Various joint of the body may be expressed as a series of links
o Each joint has a single axis
o The center of gravity for each body segment is located along the line extending from one joint to the other.
o The upper body, including the arms, may be expressed as a single, uniform volume.
o W1, f1, W2, f2, f0 are defined as follows, according to the report by Matsui: W1, 56% of body weight; f1, 45% of sitting height; W2, 10% of body weight; f2, 58% of femur length; f0, actual measured distance from the outer knee joint to the greater trochanter of the femur.
4 Conclusions
- Motion capture is an excellent tool for estimating the direct and inverse kinematics of our system; however, this procedure should be standardized, by parameterizing measures and environmental conditions where it is recorded. Once the recording data is made, it should be consider the ankle as a fixed point throughout the sitto-stand process, allowing this, a better data record.
- The Lagrange equation and Denavit-Hartemberg representation let us parameterize the kinematic analysis (position, velocity and acceleration) and dynamic (Forces and Toques) versus time, achieving these, the calculus of maximum torques and forces on each element of our model, by noticing that we can consider that we need 1N-m for each kilogram of user’s mass to get manage the sit-to-stand movement. However, it is necessary to apply an additional safety factor when selecting the actuator motor to be used in our exoskeleton.
- Newton-Euler formulation and Lagrange-Euler show similar results and graphics, corroborating thus the dynamic and kinematic analysis are correct.